ダミー変数を積極的に使用: Sample 1: 基本(C)
■ 概要
このサンプルでは式(1)にしたがって配列θから配列xとyを計算します。式(1)には式が2つあり、それぞれsin関数を使用している。そのままコーディングすると、重い演算であるsin関数を2回やることになる。しかし式(1)をよく観察すると、sin関数の引数はいずれの式も同じです。よってsin(θ)の計算結果をダミー変数にとっておけば、sin関数の実行を1回だけで済みます。
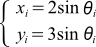
... 式(1)
下の「回答例」ボタンをクリックすれば、式(2)に回答例が表示されます。興味がある方はクリックする前にぜひ考えてみてください。

... 式(2)
Code 1は式(1)によってコーディングしたものです。Code 2は式(2)によってコーディングしたものです。配列xとyのサイズnは10,000とします。計算時間が非常に短いので、より正確な計算時間を得るためにこの計算は10,000回まわします。
■ ソースコード
|
◆ Code 1
|
◆ Code 2
|
#include <stdio.h>
#include <stdlib.h>
#include <time.h>
#include <math.h>
int main()
{
int i,j;
int n = 10000, m = 10000;
// Initialization
double *theta = new double[n];
double *x = new double[n];
double *y = new double[n];
for (i=0; i<n; i++) x[i] = rand();
for (i=0; i<n; i++) y[i] = rand();
// Start time
clock_t time0 = clock();
// Main calculation
for (j=0; j<m; j++)
for (i=0; i<n; i++) {
//
x[i] = 2.0 * sin(theta[i]);
y[i] = 3.0 * sin(theta[i]);
}
// Finish time
clock_t time1 = clock();
// Output time
double time = (double)(time1-time0)/CLOCKS_PER_SEC;
printf("Time = %15.7f sec\n", time);
delete[] theta;
delete[] x;
delete[] y;
return 0;
}
|
#include <stdio.h>
#include <stdlib.h>
#include <time.h>
#include <math.h>
int main()
{
int i,j;
int n = 10000, m = 10000;
// Initialization
double *theta = new double[n];
double *x = new double[n];
double *y = new double[n];
for (i=0; i<n; i++) x[i] = rand();
for (i=0; i<n; i++) y[i] = rand();
// Start time
clock_t time0 = clock();
// Main calculation
for (j=0; j<m; j++)
for (i=0; i<n; i++) {
double d = sin(theta[i]);
x[i] = 2.0 * d;
y[i] = 3.0 * d;
}
// Finish time
clock_t time1 = clock();
// Output time
double time = (double)(time1-time0)/CLOCKS_PER_SEC;
printf("Time = %15.7f sec\n", time);
delete[] theta;
delete[] x;
delete[] y;
return 0;
}
|
■ 計算時間の測定結果
Code 1とCode 2の計算時間の測定結果を表1に示します。ここではそれぞれのコードを5回実行して、平均とCode 1とCode 2との計算時間の比率も表示します。
表1 計算時間の測定結果(単位: sec)
|
1回目 |
2回目 |
3回目 |
4回目 |
5回目 |
平均 |
倍率 |
| Code 1 |
4.711 |
4.742 |
4.773 |
4.664 |
4.648 |
4.708 |
1.96 |
| Code 2 |
2.449 |
2.402 |
2.371 |
2.402 |
2.356 |
2.396 |
- |
■ 考察
Code 1はCode 2に比べsinの計算が2回多いため、計算時間も1.96倍遅いという結果が得ました。
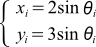 ... 式(1)
... 式(1)
 ... 式(2)
... 式(2)
